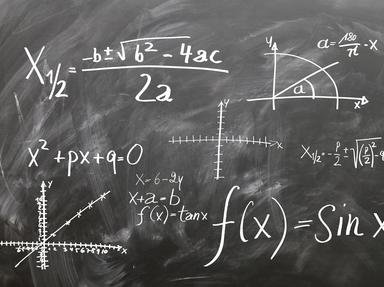Quiz Answer Key and Fun Facts
1. The quadratic equation is seen as a symbol of how oppressed school students are. It is the first equation of a certain degree of complexity faced by youngsters and, for many, the torturous equation stays with them deep into adulthood as a reminder of how awful maths was. However, quadratic equations really do, rather did, have legitimate uses. One such example is in ancient agriculture whereby farmers would calculate how much of a crop could be grown in their field. Simultaneously, it would help them to also know how much extra they needed to grow to satisfy the demands of the taxman! In which civilisation was this undertaken?
2. Quadratic equations are the basis for a vast area of more complex mathematics, both pure and applied. One such quadratic equation results in a value of "x" that is known as the "golden ratio". This ratio is represented by the letter phi and, like the representation of a lot of other quirky mathematical bits and bobs, is from a particular alphabet. Which?
3. The golden ratio has been used by many architects in their structural plans for some famous buildings. Reportedly, which of the following European buildings has the golden ratio within its dimensions?
4. The quadratic equation is of great importance in Newtonian mechanics. Quadratics are used in many equations which have everyday uses in society. Such equations that include 'v = u + (at)' and 's = (ut) + [a(t^2)]/2' can be used for what?
5. Whilst the quadratic formula seems dull and abstract for schoolchildren it has exciting applications in the science of ballistics. The fundamental force in ballistics has a value of 9.81 metres per second, per second (ms-2) and is known as what?
6. The Bernoulli effect is a physical phenomenon that originated from the humble quadratic equation. Here is another burdensome equation: (u^2/2) + P = h. This equation is helpful in which particular field?
7. Mathematics is at the very heart of physical science and this unparalleled relationship has produced great elegance which includes Schrödinger's equation. In which of these fields is Schrödinger's equation of great importance?
8. One of the most iconic sequences in mathematics is: 1, 1, 2, 3, 5, 8, 13, 21, 34, 55, 89... ad infinitum. As you can see, each value is obtained from the addition of the two preceding values (1 + 1 = 2, then 1 + 2 = 3 etc.). This sequence is useful in finance, but after which mathematician is the sequence named?
9. Calculus is a branch of mathematics established independently by Sir Isaac Newton and Gottfried Leibniz and is probably the discipline perceived as the most abstract. However, there are practical applications to this interesting area of mathematics, including medicine and pharmacology. During medical scanning, radioactive isotopes are used, so, how is this linked to mathematics and calculus?
10. Surely logarithms must be useless, right? Well in fact, they are extremely useful. One application of a logarithm is found in chemistry in the form of the mathematical expression, -log10([H+]). What does this expression help someone solve?
Source: Author
jonnowales
This quiz was reviewed by FunTrivia editor
crisw before going online.
Any errors found in FunTrivia content are routinely corrected through our feedback system.