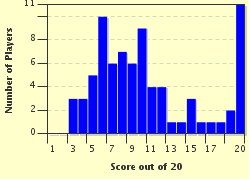
Quiz Answer Key and Fun Facts
1. Arithmetic
What is 0.166666... as a fraction?
2. Algebra
For what value of y does 3y - 8 = 21y + 28?
3. Geometry
How large is each interior angle of a 15-sided polygon?
4. Trigonometry
For what values of x, in degrees, does 2cos^2(x) - cos(x) = 0 from 0 to 360?
5. Real Functions
What is the equation of the vertical asymptote of the hyperbola y = 2/(x-3)?
6. Linear Functions
If two lines are perpendicular, then the product of their gradients is equal to what?
7. Introductory Calculus
What is the derivative of the function y = (2x+3)(5x^2-3x+1)?
8. Quadratic Function
If the quadratic ax^2+bx+c is positive definite, then which statement about its discriminant and leading coefficient is correct?
9. Locus and the Parabola
What is the equation of the directrix of the parabola (x-4)^2=16(y-3)?
10. Indices and Logarithms
What is the value of log 9, base 2?
11. Series and Sequences
For what value of n is the sum of the first n terms of the arithmetic series 2+11+20+29+...
equal to 618?
12. Financial Mathematics
If $2000 is invested at 12% p.a for 6 years, how much will be in the bank if interest is paid monthly?
13. Geometrical applications of the derivative
If a curve has a horizontal point of inflexion, then which statement is correct?
14. Integration
Find the volume of the solid of revolution formed when the curve x^2+y^2=9 is rotated about the x-axis between x=1 and x=3.
15. Trigonometric Functions
What is the derivative of y=sin^5(x)?
16. Exponential Functions
Find the exact area enclosed by the curve y=e^(3x), the x-axis and the lines x=0 and x=2
17. Logarithmic Functions
Find the gradient of the normal to the curve y=ln(x^3-5) at the point x=2.
18. Exponential growth and decay
The number of bacteria in a culture is given by N=Ae^(kt). If 6,000 bacteria increase to 9,000 after 8 hours, find when the number of bacteria will reach 1,000,000 (to the nearest hour).
19. Particle Motion
The velocity of a particle is given by v=3t^2+2t+1. If initially the particle is 2cm to the left of the origin, find the displacement of the particle after 5 seconds. (in cm)
20. Probability
If I buy 5 tickets in a raffle in which 95 tickets are sold, what is the probability that I win both the first and second prizes?
Source: Author
dialga483
This quiz was reviewed by FunTrivia editor
WesleyCrusher before going online.
Any errors found in FunTrivia content are routinely corrected through our feedback system.