Got Coordinates? Trivia Game | Math

Quiz Answer Key and Fun Facts

1. Any coordinate system worth its salt must tell you where certain points are located relative to each other, so they tend to share a few strategies. To measure the distance between points, the space is often divided with axes, lines in each dimension that define the grid. And to give the absolute position of a point, there has to be a zero-point to define the numbering scheme. Suppose I'm standing at that point, where each of my coordinates is zero. Where am I?

Answer: The origin

Take, as an example, a two-dimensional graph on a sheet of paper. The y-axis runs up the page, the x-axis runs left-to-right across the page, and the point where they intersect is the origin: (0,0). Subdivide the axes into some kind of units, and you now have everything you need to find a point on the graph. For example, you can get to the point (3,2) by moving three units right of the origin along the x-axis, and two units up from the origin parallel to the y-axis; (-3, 2) has the same y-position, but is three units left of the y-axis instead of three units right. Each axis divides the space in half, and the origin divides each axis in half.

The origin - often referred to by the letter O - may seem like a very special point, but in Euclidean geometry there's no reason it has to be located in any particular place. You can draw your axes and origin anywhere you want, as long as the axes are perpendicular to each other, and still end up with the same math. Of course, sometimes there's one point that's particularly convenient to choose as an origin, and we'll consider some of these situations later in the quiz.

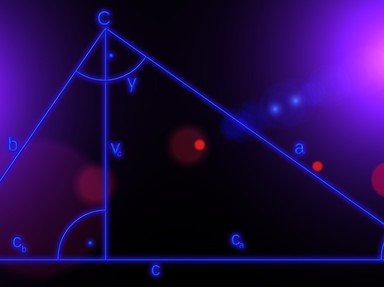
2. The first coordinate system a budding scholar learns is also the simplest. Well-suited for drawing on graph paper, it consists of just two axes meeting at right angles: x goes left and right, y goes up and down, and positions are measured on the resulting grid. This system is named after what French philosopher, who famously thought and therefore was?

Answer: Ren� Descartes

Descartes (1596-1650) was a man of prodigious ability and varied interests: he is regarded as a founder of both modern philosophy and analytic geometry. His mathematical contributions were simple but powerful: for example, he's the one who devised the modern system of writing exponents as superscripts.

There are two versions of his Cartesian coordinate system: the plane version, so easily drawn on a sheet of paper, and the spatial version, which adds a third axis (z) extending into and out of the page, at right angles to the other two. Cartesian coordinates provide a straightforward way of making numerous geometrical calculations, such as finding the slope of a line or the distance between two points.

3. There are many types of coordinate systems: some are very general, and others are more specialized. Often, a coordinate system is best suited for examining some geometry with a particular property: that it looks the same when it's rotated about some axis, say, or when it's reflected across a line. What is this type of property called?

Answer: Symmetry

There are many types of symmetry. For example, the letter T has a reflection symmetry with a vertical axis, which means that, if you divide it vertically, the two halves are mirror images of each other. The letter T doesn't have a horizontal symmetry axis, but the letter E does. Meanwhile, the letter O has rotational symmetry: it looks the same no matter what angle you rotate it by. And all the letters have translational symmetry: they look the same no matter where they appear on the page.

4. A basic, grid-type coordinate system works well for studying shapes that are made up of straight lines, but it is often inelegant when describing curves. A better coordinate system for these purposes is one where a point is specified by a linear distance and an angle, rather than by two linear distances. What is this curvilinear system called?

Answer: A polar coordinate system

A polar coordinate system is defined by a single point (the origin) and a single line (the polar axis). A location is specified by its distance from the origin (usually denoted by the letter r, for radius) and by its angular separation from the axis (usually denoted by the Greek letter theta, which looks like a 0 with a horizontal line through the middle). By convention, the polar axis is drawn as a horizontal line starting at the origin and extending to the right, and the angle theta is measured counter-clockwise. The polar coordinates of a given point are not unique -- adding 360 degrees to the angle gets you back where you started.

This may seem an awful lot of work, but polar coordinates allow for very elegant descriptions of many shapes. For example, you can draw a spiral from the equation r=theta, and a flower from r=2*sin(5*theta). Beautiful!

5. So far, we've looked at coordinate systems that help us plot what's happening on a planar surface, like a sheet of graph paper or a map -- but this just isn't enough for understanding most real-world objects. If we want to examine volumes, not just areas, what must we add to any planar coordinate system?

Answer: A third dimension

The surfaces of maps and sheets of graph paper exist in two-dimensional space: they can be described completely by considering only two dimensions which are called east-west and north-south on a map. But we live in a world with three spatial dimensions; what about up-down? This third dimension is hard to represent on a two-dimensional surface, but the laws of perspective let us make plots that give a sense of it -- and we can always use an appropriate three-dimensional coordinate system to study the mathematical properties of a three-dimensional object.

6. One way of plotting the coordinates of a volume involves two distance coordinates and an angular coordinate. One distance is measured along a central axis, and the other is measured perpendicular to that axis. The angle specifies a point on the circle swept out by the second distance variable. What type of shape is this coordinate system best suited for describing?

Answer: A cylinder

The cylindrical coordinate system is basically polar coordinates plus. The radial distance r and the angle theta specify a point on a two-dimensional circle centered on an axis, and the distance z specifies the position of the circle's center along that axis, adding a third dimension in the most straightforward possible way.

A coordinate system like this is tailor-made for describing situations with cylindrical symmetry: the layouts of pipes, for instance, or the electromagnetic field produced by a current on a wire.

7. Other types of volumes are best described with a spherical coordinate system, which features two angular coordinates and one distance coordinate. We use a variation on this system to locate points on the Earth's surface: the distance from the center is just the altitude added to the radius of the Earth. What do geographers call the two angular coordinates?

Answer: Latitude and longitude

Imagine cutting the sphere of the Earth in half at the equator to get a circular cross section. Measuring the angle from the Prime Meridian gives you the longitude of any point on the circle, from 0 to 360 degrees. A similar trick lets you visualize the other angle: if you cut the Earth in half the other way, slicing north to south, you can see that every line of latitude is specified by an angle from 0 to 90 degrees on either side of the equator. If you've ever wondered why latitude and longitude are measured in degrees, this is why!

More generally, spherical coordinate systems designate the radial distance (the distance out from the origin) by the letter r or by the Greek letter rho, which resembles a p. The angles are represented by the Greek letters theta (a 0 with a horizontal line through it) and phi (an o with a vertical line through it). Unfortunately, mathematicians and physicists disagree as to which letter corresponds to which angle, which can make for some confusing moments if you're consulting one of each type of textbook.

8. Suppose that you're making a graph whose values span a huge range - there's interesting structure at 1, at 10,000, and at 1,000,000! To display all of this clearly, we can use a coordinate scale where the distance between two points on an axis is defined by their ratio - not by their difference. What do we call this type of scale?

Answer: Logarithmic scale

A logarithm is a mathematical function for dealing with exponents. Say the base-x logarithm of y is equal to n; that means that x raised to the nth power, or x^n, is equal to y. This is a fantastically useful algebraic tool, but it's also handy when it comes to cramming a wide range of information into a compact graph. On an axis with a linear scale, 1 and 2 are the same distance apart as 2 and 3; on an axis with a logarithmic scale, however, 1 and 10 are the same distance apart as 10 and 100, because each pair of ticks is a factor of 10 apart - a constant ratio.

Although this may seem arcane, you're probably familiar with the system from a few famous examples. The Richter scale, which measures the strength of earthquakes, is a logarithmic scale: an earthquake that registers a 7 on the Richter scale is ten times as severe as an earthquake that registers a 6. And logarithmic scales are natural, too; studies have shown that our hearing works in a logarithmic scale. We hear the same pitch difference between a frequency f and a frequency 2f as we do between 2f and 4f!

9. Coordinate systems are useful for describing shapes, plotting relationships between variables, and performing various mathematical calculations (like finding the volume of a cone). Yet they can also be useful for exploring more abstract concepts. One such system is the complex plane, which allows mathematicians to plot complex numbers of the form a+bi. What does i mean, mathematically?

Answer: i is the square root of -1.

In mathematics, every number has a square root, negative numbers included. The letter i denotes the archetypal "imaginary number," the square root of -1; by contrast, "real numbers" yield non-negative values when squared. All complex numbers can be expressed in the form a+bi, where a and b are real numbers (and could be equal to zero). The complex plane provides a nice way of plotting complex numbers as pairs of coordinates: a gives the position on the horizontal axis, and b gives the position on the vertical axis.

Complex numbers can seem arcane, but they actually open up exciting areas of mathematics. For example, the trignometric functions sine and cosine can be expressed by summing complex numbers, and i turns up everywhere in quantum mechanics.

10. It isn't only humans who need to find themselves: how do you tell which star is which unless you know where they are? Astronomers use a system called equatorial coordinates, which essentially maps longitude and latitude lines onto the inverted bowl of the sky. But in addition to latitude and longitude, they must also specify what time period, or "epoch," their coordinates are valid for. Why is this?

Answer: Since the Earth rotates like a top, our axis points in different directions over time - changing the coordinate system relative to the stars.

The name for the astronomical equivalent of latitude is declination, which works the same way as its earthly counterpart: if the star is directly above the equator, it's at 0 degrees, and the angles go positive for northerly stars and negative for southerly ones. Right ascension, meanwhile, works much like longitude (except that it's traditionally reported in hours, minutes and seconds). Like longitude, the starting point is arbitrary; by convention, astronomers choose to measure beginning from the place where the Sun crosses the zero-degree declination line during the March equinox. (That's one of the two times per year when the equator sees an equal amount of daylight and night.)

This system is very efficient and even somewhat intuitive - until you realize that the Earth precesses. Objects can rotate different ways; for example, a bicycle wheel rotates around a fixed axis (at least while the bicycle is straight up!), but when you spin a top, it rotates around an axis that moves in the shape of a circle. The Earth rotates like a top, which is called precession; its axis won't point exactly the same way as it does now for another 25,765 years! And as our axis changes, so does our view of the stars. Astronomers must adjust their coordinate system roughly every 50 years in order to correct for this effect; after all, it's helpful if a star's coordinates tell you where you can actually find it!

Source: Author CellarDoor

This quiz was reviewed by FunTrivia editor crisw before going online.
Any errors found in FunTrivia content are routinely corrected through our feedback system.

Got Coordinates? Trivia Quiz

Finding your position in space is useful for mathematicians as well as navigators. Do you want to plot a function, draw a line or describe a solid shape? Take this quiz and exploit coordinate geometry!

NEXT>

NEXT>

NEXT>

NEXT>

NEXT>

NEXT>

NEXT>

NEXT>

NEXT>